Effects of cavitation on the radial force and pressure pulsation of a centrifugal pump
-
摘要:
不稳定空化流动会诱发离心泵内发生高幅值压力脉动并加剧泵体振动，影响其安全稳定运行。该研究以闭式离心泵为研究对象，通过非稳态数值模拟探究了设计流量下叶轮径向受力及压力脉动随空化发展的变化规律，分析了空泡变化对压力脉动的影响。研究结果表明：随着空化的发展，叶轮径向力先减小后增大，变化拐点为前盖板附近出现游离片状空化；完全空化状态下径向力呈多边形分布，大小约为无空化状态的1.4倍；不同空化状态下，叶轮径向力变化频率以转频fn及其倍频为主。压力脉动突增发生在空泡团破碎阶段；不同空化状态下，监测点压力脉动主频均为转频fn；严重空化时，空泡团的缓慢演化会在下游诱发0.5fn的低频压力脉动。研究结果可为离心泵空化监测和诊断提供参考。
Abstract:Cavitation is one kind of complex multiphase flow. Unstable cavitation flow can often induce a high amplitude of pressure pulsation, leading to the vibration of the pump body inside the centrifugal pump. A threat can also be posed to the safety and stability of pump operation. This study aims to explore the variation in the radial force and the pressure pulsation of the impeller, as the cavitation developed. A closed centrifugal pump was taken as the research object. Unsteady numerical simulation was carried out to investigate the cavitation flow in the centrifugal pump under a given flow rate. The homogeneous Eulerian-Eulerian two-fluid model was used to simulate the cavitation flow. Two-equation SST (shear stress transmission) k-ω model was adopted as the turbulence model to close the URANS (unsteady reynolds averaged navier-stokes) equations. The Schnerr-Sauer cavitation model was used to solve the volume fraction of the vapor phase. The reliability of the model and numerical simulation was verified to compare with experimental values. A systematic analysis was made to determine the influence of vapor bubbles on radial force and pressure pulsation. The research results indicated that the time-averaged radial force on the impeller first slightly decreased and then suddenly increased as the cavitation developed. The turning point was observed in the condition that the sheet cavitation appeared near the shroud. Furthermore, the time-averaged radial force reached as high as 60.5 N under complete cavitation, which was 1.4 times higher than under non-cavitation. And the peak value of radial force reached the maximum of 120 N, which was 1.69 times that of no cavitation stage. The high amplitude radial force caused the pump body more prone to instantaneous large vibration. The presence of bubbles disrupted the symmetry of pressure distribution, resulting in a polygonal distribution of radial force. The frequency of radial force variation was dominated by the impeller rotational frequency and its multiplication under different cavitation states. Once the cavitation was developed to the critical value, there was no variation in the amplitude of radial force corresponding to the impeller rotational frequency, while the amplitude of its multiplication increased only. When the net positive suction head was 1.9 m, the amplitude of radial force corresponding to the impeller rotational frequency was 41 N, which was about 1.4 times that under non-cavitation. The amplitude of radial force was 10 N corresponding to the sub-frequency of 2 times the impeller rotational frequency, which was 1.7 times that under non-cavitation. The influence of the breaking of vapor bubbles on pressure fluctuations shared a certain degree of global significance. This influence also depended on the relative position of the monitoring points and bubble clusters. The pressure pulsation coefficient and standard deviation were basically 0 when the monitoring point was located inside the bubble cluster. There was a significant increase and then a decrease, as the vapor bubbles were broken and aggregated when the monitoring point was located near the bubble clusters. The pressure wave generated by the breaking of vapor bubbles was also found downstream along the flow direction. The main frequency of pressure pulsation at each monitoring point was the impeller rotational frequency under different cavitation. The slow evolution of bubble clusters in the impeller induced the low-frequency pressure pulsations of 0.5 times the impeller rotational frequency during the complete cavitation. Consequently, the main frequency amplitude of radial force in the pressure pulsation was more suitable for evaluating the development of the cavitation flow field. The findings can also provide theoretical references to monitor and identify the cavitation in centrifugal pumps.
-
Keywords:
- centrifugal pump /
- cavitation /
- numerical simulation /
- pressure fluctuation /
- radial force
-
0. 引 言
离心泵因结构简单紧凑、扬程大等优点被广泛用于工农业生产中[1-2]。实际运行过程中，由于运行环境及工况的变化，离心泵进口低压区易发生空化流动[3-5]。空泡的产生不仅会堵塞流道，造成泵性能下降，同时空泡的破裂和聚合会造成流场不稳定，诱发振动和噪声，给设备和系统运行带来安全隐患[6-8]。
径向力指作用于叶轮径向方向的力，径向力过大是造成轴承与密封磨损的主要原因[9]。叶轮径向受力不平衡的原因一般有质量偏心、安装误差和流动不稳定等[10]。 叶轮内发生空化后，各流道空泡分布往往存在差异，导致叶轮内各流道流量出现偏差，从而诱发不平衡径向力。加之空泡破碎时产生的强烈冲击波作用于叶轮表面，造成材料剥落，导致叶轮质量发生偏心，从而进一步加剧叶轮径向受力的不均。ADAMKOWSKI等[11]研究发现，空化的存在虽然并未使泵内的压力脉动发生特殊变化，但空蚀的出现使得转轮质量出现明显不均，破坏了转动的平衡，从而诱发系统与轴的共振，并最终造成转轴断裂。而且空泡破碎产生的冲击波会诱发瞬时高压，导致泵体振动加剧。因此研究空化引起的不稳定流动及其对水力机械运行稳定性的影响一直以来都是研究人员关注的重点[12-17]。吴登昊等[18]发现不同流量下叶轮内空泡分布存在明显差异，并指出空泡的存在会破坏径向力的分布。ZHU等[19]分析了不同流量下空化引发的离心泵轴向受力变化，并通过载荷分析揭示了空化导致轴向力变化的原因。李琪飞等[20]发现随着空化的加剧，叶轮所受径向力的对称性和周期性被破坏。HAO等[21]发现空化不仅会增大非对称叶顶间隙下的径向力，还会改变其方向。GONG等[22]发现泵运行在临界空化状态时，大尺度空化云团的塌陷会导致监测点出现大幅度的瞬态压力冲击，进而加剧X方向的振动幅度。高波等[23]和叶阳辉等[24]也在试验中发现空化会加剧泵体振动。JIA等[25-26]通过数值模拟也发现空化会导致叶轮径向力显著波动，并破坏其对称性和连续性。
综上所述，空化的产生会导致叶轮径向受力改变，并加剧机组振动，对泵的安全稳定运行造成威胁。因此，深入了解空化条件下的叶轮径向力及内部压力脉动的变化规律及产生机理，对于指导离心泵运行和设计，延长泵的使用寿命，降低维护成本有重要意义。本文以一闭式离心泵为研究对象，分析叶轮受力和压力脉动随空化程度的发展变化规律，明确空泡演变与压力脉动和径向力之间的关联，以期为改善空化造成的离心泵运行不稳定问题提供依据。
1. 数值模拟方法
1.1 几何模型
本文离心泵试验数据和几何模型源于文献[27-28]。研究对象由进口管、前后泵腔、叶轮和无导叶扩压器4部分组成，如图1所示。扩压器出口管段的连续90°折弯会引起过大的水力损失，因此在直径
1000 mm处沿圆周方向布置8个监测点，并采用监测点处的总压作为出口压力计算泵的扬程[27]。该泵主要运行参数如下：设计流量Qd = 412 m3/h；额定转速n = 540 r/min；设计扬程Hd = 10.16 m。其余运行及几何参数见参考文献[27]。1.2 网格划分
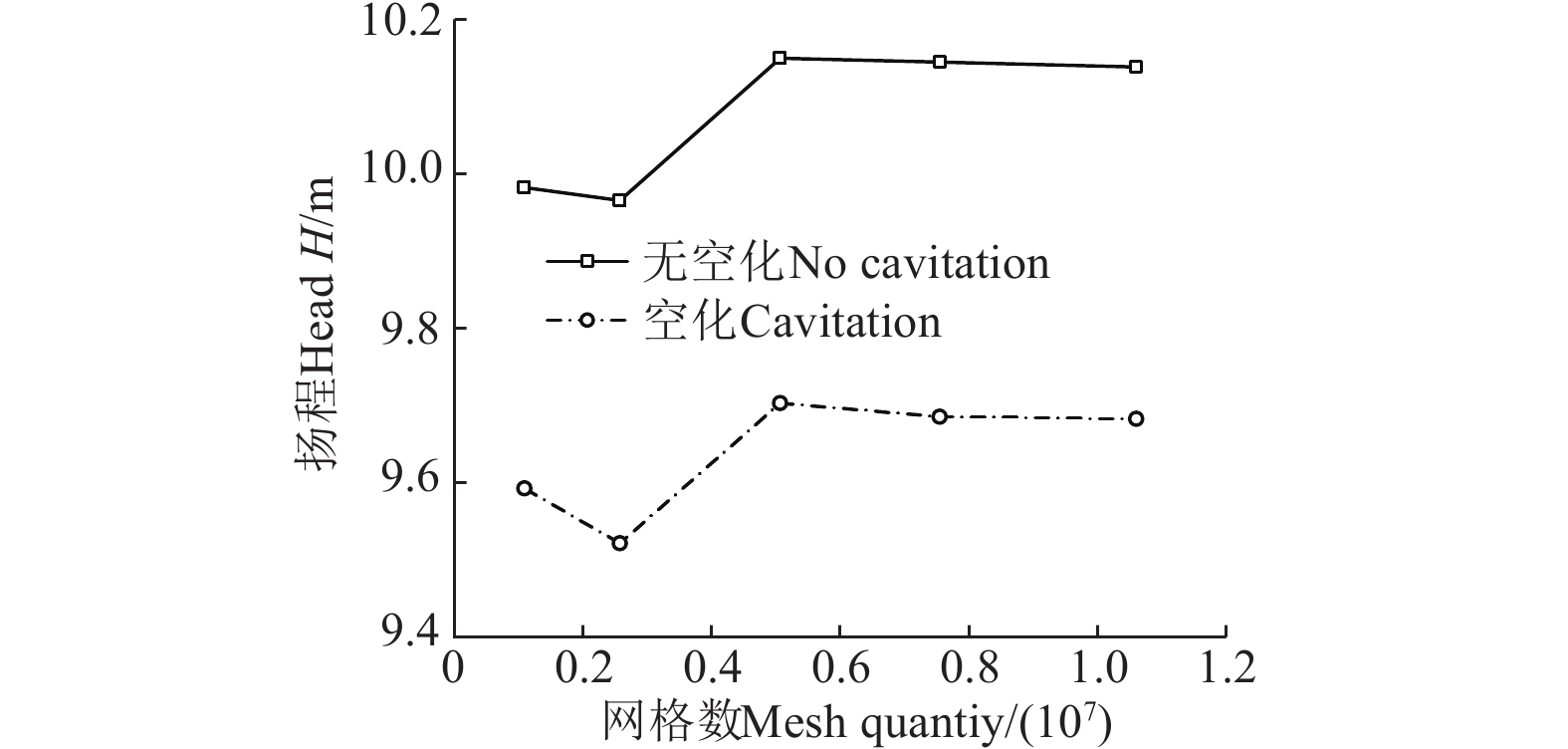
对各过流部件进行结构化网格划分，并对近壁面区域进行局部加密以保证湍流模型在近壁面区域的适用性。为降低网格数量带来的计算误差，节省计算资源，选取5套不同数量的网格分别对无空化条件下设计点和空化条件下装置空化余量为2 m的工况进行网格无关性验证。从图2发现当网格数量大于754万后，泵扬程波动小于1%。因而选取网格总数为754万的第四套网格方案作为最终计算网格，网格结构如图3所示。
1.3 计算设置
进口给定为压力进口，单相计算时压力设置为101 325 Pa，空化计算时进口压力由式（1）确定，出口给定为质量流量。空化计算时介质选择25 ℃的水和水蒸汽。两相流模型选择均相流模型，不考虑相间传热。传热模型设置为绝热等温，壁面为无滑移光滑壁面。湍流模型选择SST k-ω模型[29]，空化模型选择Schnerr-Sauer模型[30]。非定常计算以定常计算结果为初值，计算时长为1.666 7 s（叶轮转动15圈）。收敛残差设置为平均残差小于10−5。为了平衡计算资源和计算准确性，时间步长选择叶轮每转过2°的时长为一步，以计算稳定后最后5圈的结果作为最终结果。
NPSHa=Pin−Pvρg (1) 式中Pin为进口总压，Pa；Pv为饱和蒸汽压，3 574 Pa，NPSHa为装置空化余量，m，ρ为密度，kg/m3；g为重力加速度，m/s2。
1.4 试验验证
为了保证数值模拟的准确性，将纯水和空化条件下泵的外特性模拟值与试验值进行对比，如图4所示。纯水工况下模拟获得的外特性基本与试验值接近，整个流量范围内扬程误差在4%以内，效率误差在2%以内。空化条件下数值模拟得到的H-NPSHa曲线虽略滞后于试验值，但两者的变化趋势基本一致，除严重空化点外，其余工况下扬程曲线误差在6%以内。考虑到空化流动的复杂性，该结果可接受，本文采用的模型和计算设置合理可靠。
2. 结果与分析
2.1 叶轮径向受力随空化发展的变化规律
空泡的出现破坏了叶轮内流场的对称性，是造成径向力变化的主要原因，图5给出了5个周期下叶轮内空泡体积Vc和径向力ˉFr随NPSHa的变化曲线。其中时均径向力ˉFr计算式如下：
![]()
图 5 时均径向力 ˉFr 和叶轮内空泡体积Vc积随NPSHa的变化Figure 5. Variation of time-average radial force ˉFr and volume of cavity Vc in impeller with NPSHaˉFr=1NN∑i=1Fri (2) 式中N为数据点个数，900；Fri为瞬时径向力，N。
随着NPSHa的减小，叶轮内空泡体积先大致保持平稳，在NPSHa 值小于2.1 m后急剧增加。空化初生阶段，少量汽泡的产生改善了各流道内流量分布不均的现象，同时附着的空泡减弱了来流对叶片头部的直接冲击，从而使得ˉFr在该阶段出现小幅下降。当NPSHa 值小于2.5 m后，叶轮径向力逐渐增大。严重空化时(NPSHa = 1.8 m)，ˉFr高达60.5 N，约为无空化工况(NPSHa = 4.5 m)的1.4倍。对比径向力与空泡体积变化的趋势，两者发生突变位置不仅存在差异，且在一定程度后变化也不再相似，这说明影响叶轮内空泡体积的增大并非诱发径向力变化的根本原因，需要进一步分析。
图6为2个旋转周期(T)内叶轮径向力的时域和频域分布。一个周期内转轮径向力的曲线并不完全光顺，出现多个小范围的锯齿形波动，反映了流场扰动对径向力的影响。对比不同NPSHa下径向力的分布可以发现，除NPSHa = 1.8 m外，其余工况下径向力的时域分布类似，仅幅值存在一定差异。NPSHa = 1.8 m时，一个周期内径向力变化复杂，且此时径向力峰值明显增大，最高达120 N，为无空化状态的1.69倍。高幅值径向力使泵体更易发生瞬时大幅振动。
![]()
图 6 叶轮径向力时域和频域分布注：T为旋转周期，0.111 1 s；fn为转频，9 Hz；f为实际频率，Hz。Figure 6. Time and frequency domain distribution of radial forceNote: T is the rotation period, 0.111 1 s; fn is rotating frequency, 9 Hz; f is the actual frequency, Hz.叶轮所受径向力主要以转频fn及其低倍频为主。NPSHa = 4.5 m时主频fn对应的径向力为28.9 N。当NPSHa = 2.5 m时，该值减小到25.7 N。随后该值开始增大。但NPSHa小于2 m后，主频fn对应的径向力不再变化，保持在41 N左右，约为无空化条件下的1.4倍。此后次主频对应的径向力增大。NPSHa = 1.9 m时，次主频2fn对应的径向力为10 N，为无空化条件下的1.7倍。当NPSHa = 1.8 m时，径向力的次主频由2fn变为4fn。对比不同NPSHa下的径向力的频域信息发现，空化主要影响径向力的大小，而对径向力的主要频率影响很小。
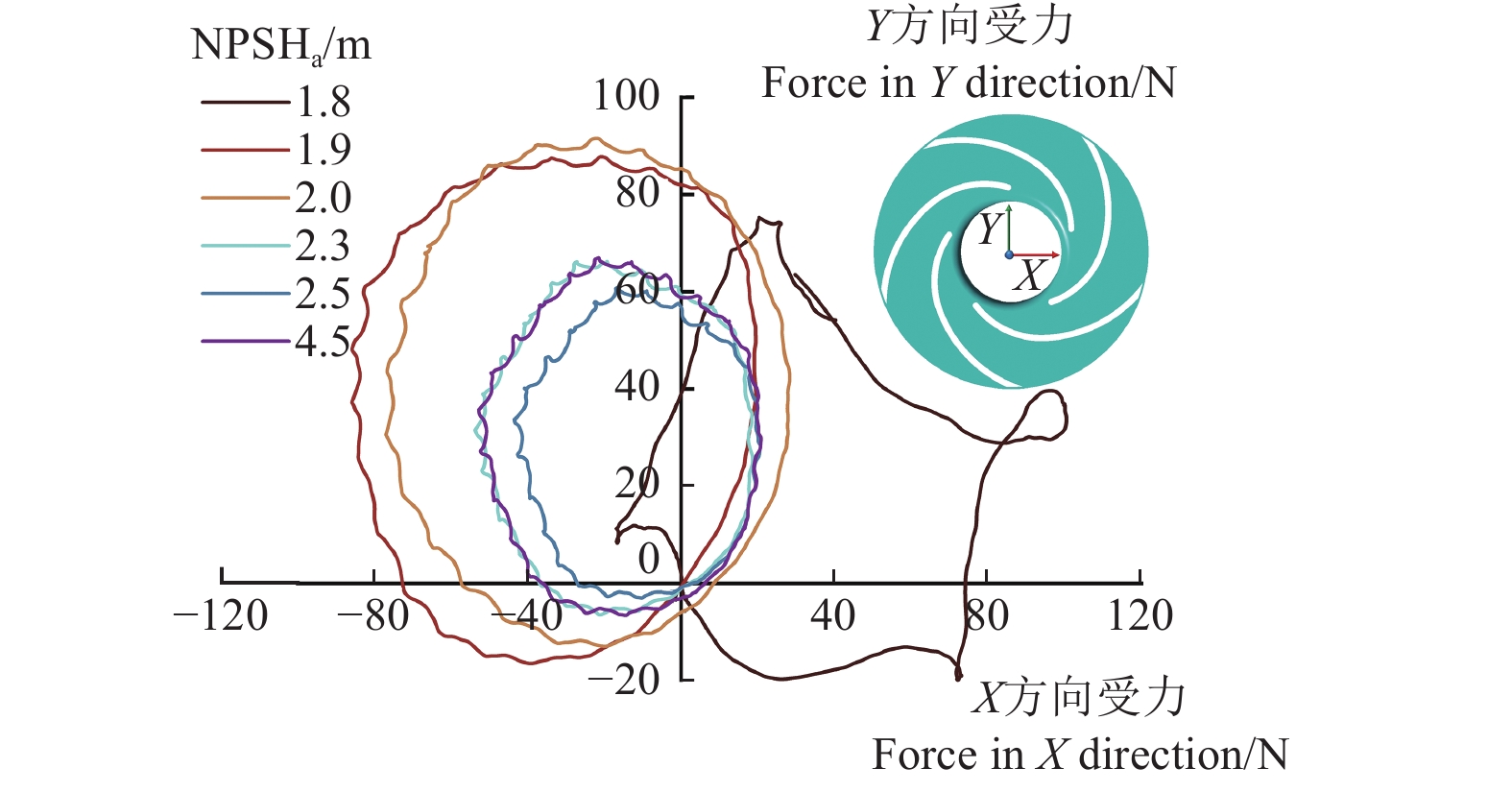
图7为一个周期内X，Y方向的叶轮受力分布。图中各点坐标与原点的距离表示径向力的大小，相对原点的位置代表径向力的方向。由于本文研究对象采用无导叶扩压器，从图中可以看出，除NPSHa = 1.8 m的工况外，其余空化条件下叶轮在X，Y方向的受力基本呈椭圆形分布，且椭圆的大部分位于第二象限。这表明进入到叶轮5个流道内的流量并非完全相同，各流道之间存在一定的差异。NPSHa 小于 2.5 m后，空泡会加剧这一不均匀性，使得径向力沿偏心方向增大。完全空化状态时（NPSHa = 1.8 m），叶轮内空泡团分布更为复杂，叶轮径向力呈不稳定多边形分布，方向由第二象限偏向第一象限。
出口压力沿周向分布的不均，是造成叶轮径向力的主要原因[9]，图8为叶轮出口压力的分布。可以看出，叶轮出口处静压基本呈周期性分布，而空化并不会破坏这种周期性，而且由于本文中叶轮径向力本身相对较小，因此很难完全通过出口压力分布判别径向力的变化。分析叶轮径向力频域图可知，叶轮径向力主要以转频及其低倍频为主，这种情况可以认为是由于转轮内周向质量不均匀导致的，因此本文进一步对各流道内的质量流量分布进行分析。为了方便讨论转轮各流道内质量流量的变化，定义无量纲质量流量系数m如下：
m=Qmi5∑i=1Qmi (3) 式中Qmi为各流道质量流量，叶轮无量纲质量流量系数分布见图9。
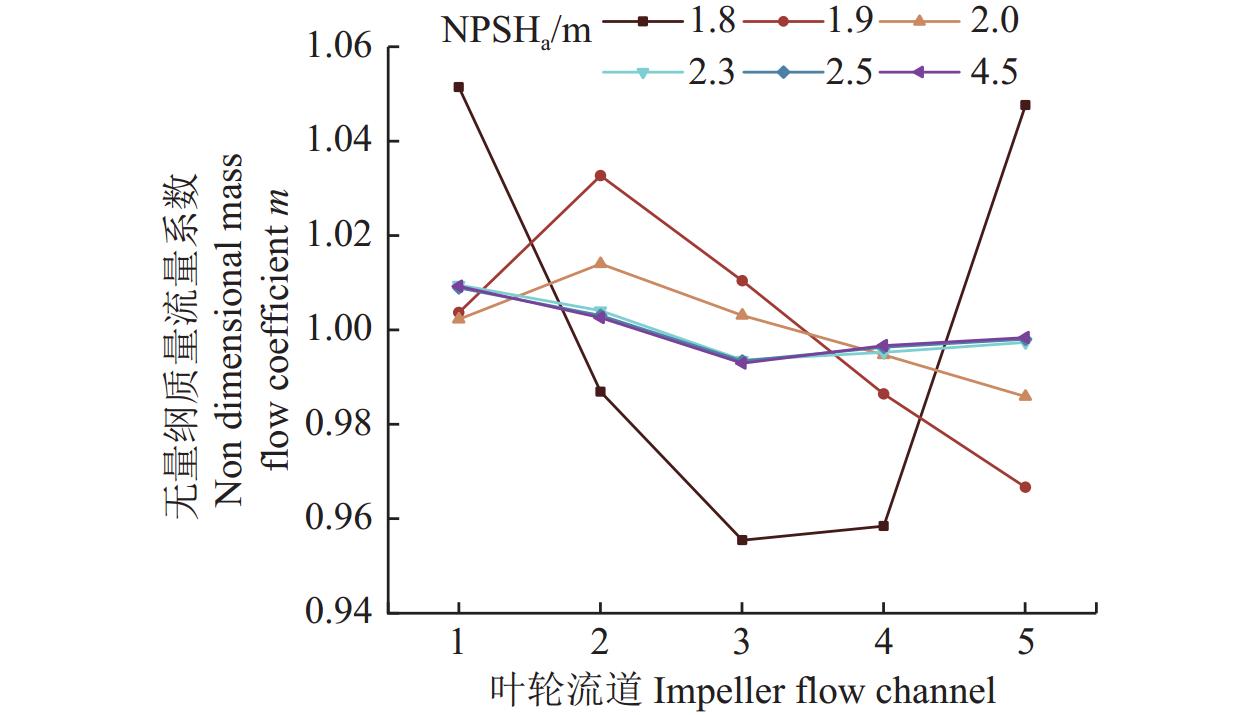
从图9中可以发现，NPSHa ≥ 2.3 m时，各流道内m的分布状态基本接近，所以其径向力变化相对较小。而当NPSHa < 2.3 m后，片状空化的迅速发展，使得叶轮各流道内部质量流量均匀性被破坏，叶轮2、3流道质量流量较大，而4、5流道质量流量减小。但此时大质量流量仍集中1、2、3流道，故径向力方向仍未发生偏转。NPSHa = 1.8 m以后，质量流量分布的不均性进一步增大，此时2、3、4流道内质量流量减小，而1、5流道质量流量增大，径向力方向此时也发生改变。
质量流量分布的不均，一般主要由空泡形态的差异所引起。图10为空泡体积分数αv = 0.1时不同空化状态下叶轮内的空泡形态。空泡首先呈条状附着在叶片头部吸力、压力面。并随着NPSHa的减小，吸力面空泡向下游发展，形成薄片状空化。当NPSHa减小到2.3 m时，吸力面空泡不再增长，此时叶轮进口靠近前盖板处出现游离的片状空化。随后游离的片状空化迅速发展，在前盖板处形成超空化，而此时叶轮内各流道质量流量分布不均性也逐渐明显。NPSHa = 1.8 m时，前盖板处超空化现象进一步发展，同时叶片吸、压力面空泡团也飞速增长，加剧了叶轮内空泡分布的复杂性。这使得叶轮内周向质量流量的分布完全被破坏，从而造成径向力分布的改变。
![]()
图 10 不同工况下叶轮内空泡形态及分布（空泡体积分数αv = 0.1）Figure 10. Morphology and distribution of cavity inside impeller under different NPSHa (volume fraction of vapor αv = 0.1)2.2 压力脉动随空化发展的变化规律
为分析空化流动诱发的不稳定压力脉动现象，沿流向在近前盖板靠近吸力面的位置布置4个监测点，其中P1，P2点位于空泡团附近，P3，P4点位于空泡团下游，如图11所示。为了方便对比，对监测点压力做无量纲处理，无量纲压力脉动系数Cp的计算式如下：
Cp=P−Pv0.5ρlu22 (4) 式中P为局部静压，Pa；Pv为饱和蒸汽压(3 574 Pa)；ρl为液体密度，kg/m3；u2为转轮出口圆周速度，m/s。
同时引入压力脉动的标准差σ表征不同空化状态下各点的压力脉动变化，其计算如式（5）所示。σ值越大，表明数据点偏离均值越明显，即压力变幅越大。
σ=√N∑i=1(Cpi−ˉCp)2N (5) 式中Cpi为压力脉动系数的瞬时值；¯Cp为压力脉动系数的平均值；N为样本数量。
图12和图13分别为压力脉动标准差σ以及压力脉动系数Cp的时域分布。NPSHa ≥ 2.5 m时，微量气泡的产生改善了叶轮流道流量分布不均的现象，各监测点压力脉动标准差略有下降。NPSHa < 2.5 m后，各点压力受空化的影响逐渐明显（σ值增大）。NPSHa = 2 m时，P1点基本位于空泡团内，其Cp值锐减，但受空泡变化的影响，压力振荡幅度略微增大（σ值由
0.0022 增大到0.0043 ）；P2点由于处于空泡团边缘，受空泡团变化的影响最明显（Cp在0～0.089间剧烈波动），标准差σ由NPSHa = 2.3 m时的0.0027 增大为0.036；P3，P4两点由于距离空泡团相对较远，空泡变化对其影响小于P2点，但σ值仍略有增大。随着NPSHa的减小，空泡团逐渐向下游发展，各监测点逐渐被空泡团占据（Cp ≈ 0），压力场也趋于稳定（σ ≈ 0）。当NPSHa减小到1.8 m时，仅P4点仍存在明显压力脉动，其余各点的压力脉动系数和标准差基本为0。![]()
图 12 各监测点压力脉动标准差及空泡体积变化加速度峰值分布Figure 12. Standard deviation distribution of pressure pulsation and peak value of acceleration change of cavity volume at monitoring points![]()
图 13 各监测点压力脉动时域分布注：t0～t5 为不同时刻，s。Figure 13. Time domain distribution of pressure pulsation at monitoring pointsNote: t0 –t5 are different times, s.对比图12中P2点由NPSHa=2.3 m变为2 m和P3点由2 m变到1.9 m时σ的变化程度可以发现，空化的进一步发展并未造成P3点压力脉动的剧烈增大。这可能主要是由于NPSHa=1.9 m时，叶轮内空泡体积变化的加速度峰值相对较小所造成的。根据文献[13]，一定空化程度后，空泡附近监测点的压力脉动与空泡体积变化的二阶导数成正比，此空泡体积变化加速度越大，附近监测点的脉动波动也越明显。如12所示，可以看出NPSHa < 2.5 m，空泡体积变化加速度峰值开始逐渐增大，尤其是由2.3 m减小到2.0 m时，发生突增，随后在1.9 m时略有下降。当NPSHa=1.8 m，该值进一步增大，这也是尽管P4点距离空泡团较远，但其σ值仍明显增加的主要原因。
图14为图13b中6个时刻对应的空泡体积分数云图。t0时刻，P2点位于空泡团内，其压力脉动系数基本为0。t1时刻空泡团开始破碎，产生瞬时高压，导致P2点压力脉动系数逐渐增大。随着空泡破碎的进一步发展，P2点的压力幅值在t3点基本达到最大。随后流道内空泡开始聚集，P2点压力脉动系数开始下降。t5时刻空泡团继续发展，此时压力脉动的幅值仍继续下降。整个过程中P1点基本位于空泡团边缘，故其Cp值很小，但标准差却略微增大。P3，P4距离空泡团有一定距离，但从图12标准差的变化来看，其仍受到了一定程度的影响，这说明空泡团的变化会对下游流场造成扰动，将压力波传递至下游。综合来看空泡对压力脉动的影响虽具有一定的全局性，但影响程度取决于监测点与空泡团的相对位置。
![]()
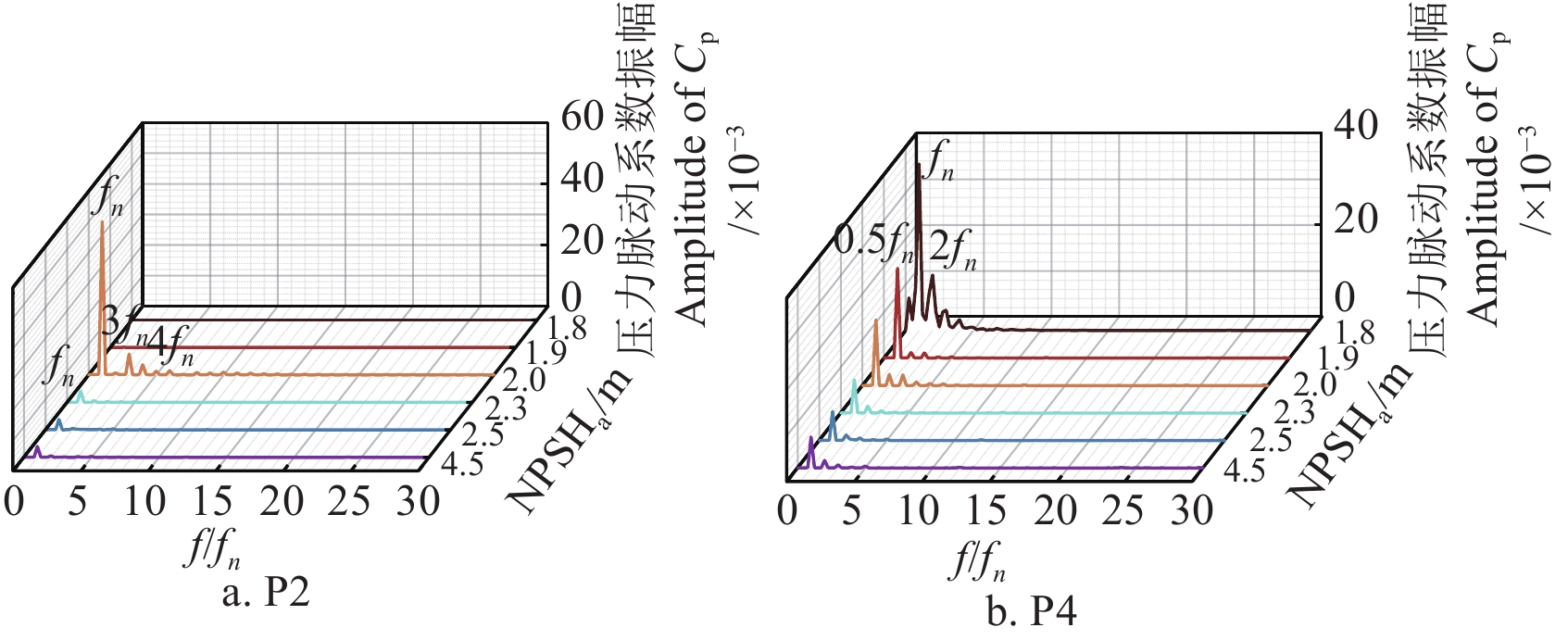
图 14 不同时刻叶轮内0.9倍叶高处空泡体积分数分布Figure 14. Distribution of vapor volume fraction at 0.9 times blade height at different times图15和图16分别为空泡体积和P2、P4两点的压力脉动频域分布。
![]()
图 16 P2和P4点压力脉动频域分布Figure 16. Frequency domain distribution of pressure pulsation at P2 and P4 points可以发现不同空化状态下各点的压力脉动主频基本以转频fn为主，而各点对应的次频在不同空化状态下略有差异，但整体仍以转频的倍频为主。NPSHa = 1.8 m时，P4点的次频中出现了0.5fn，这与空泡体积演化的主频一致。造成这一现象的原因可能是由于此时叶轮中空泡团体积较大，加之严重空化状态下叶轮做功能力减弱，压力梯度减小，回射流对空泡体积的影响减弱，导致空泡体积演化时间增长。对比空泡团与压力脉动的频域分布可知宏观空泡行为可能会影响压力脉动的频域分布，但影响很弱，空泡团主要影响各频率对应的压力脉动幅值大小。
3. 结 论
本文采用数值模拟方法探究了不同空化状态下离心泵内的流动现象，并通过分析空泡团的演变，明确空化对径向力和压力脉动的影响，主要结论如下：
1）空泡的产生会导致叶轮内周向流量分布出现差异，并诱发径向力变化。前盖板出现游离片状空化后，周向质量流量分布更加不均，径向力开始增大。严重空化工况下甚至会导致径向力方向发生改变。
2）空化主要影响叶轮径向力的大小，而对其频域分布影响较小。不同空化状态下径向力的主频和次主频均以转频fn及其低倍频为主。装置空化余量（NPSHa）小于2 m后，主频fn对应的径向力维持在41 N左右，此后径向力增大主要由次主频幅值变化引起。严重空化工况下主频fn和次主频2fn对应径向力大小可分别达到无空化条件下的1.4和1.7倍。
3）空化主要影响压力脉动的大小，对其频域分布影响较小。空泡团对压力脉动大小的影响受其体积变化加速度及相对位置双重影响。严重空化工况下，空泡团的宏观演化行为，会诱发监测点出现0.5fn的特殊低频。
研究结论有助于加深理解空化对离心泵运行稳定性的影响机理，可为实际生产中离心泵空化监测和诊断提供理论依据。
-
![]()
图 5 时均径向力 ˉFr 和叶轮内空泡体积Vc积随NPSHa的变化
Figure 5. Variation of time-average radial force ˉFr and volume of cavity Vc in impeller with NPSHa
![]()
图 6 叶轮径向力时域和频域分布
注：T为旋转周期，0.111 1 s；fn为转频，9 Hz；f为实际频率，Hz。
Figure 6. Time and frequency domain distribution of radial force
Note: T is the rotation period, 0.111 1 s; fn is rotating frequency, 9 Hz; f is the actual frequency, Hz.
![]()
图 10 不同工况下叶轮内空泡形态及分布（空泡体积分数αv = 0.1）
Figure 10. Morphology and distribution of cavity inside impeller under different NPSHa (volume fraction of vapor αv = 0.1)
![]()
图 12 各监测点压力脉动标准差及空泡体积变化加速度峰值分布
Figure 12. Standard deviation distribution of pressure pulsation and peak value of acceleration change of cavity volume at monitoring points
![]()
图 13 各监测点压力脉动时域分布
注：t0～t5 为不同时刻，s。
Figure 13. Time domain distribution of pressure pulsation at monitoring points
Note: t0 –t5 are different times, s.
![]()
图 14 不同时刻叶轮内0.9倍叶高处空泡体积分数分布
Figure 14. Distribution of vapor volume fraction at 0.9 times blade height at different times
-
[1] 刘博星,卢金玲,冯建军,等. 阀板尾迹对离心泵性能的影响[J]. 农业工程学报,2024,40(2):217-227. LIU Boxing, LU Jinling, FENG Jianjun, et al. Influence of valve plate wake on the performance of centrifugal pump[J]. Transactions of the Chinese Society of Agricultural Engineering (Transactions of the CSAE), 2024, 40(2): 1-11. (in Chinese with English abstract)
[2] 王凯,柳涵宇,王李科,等. 颗粒体积浓度对半开式叶轮离心泵泄漏涡和磨损的影响[J]. 农业工程学报,2023,39(16):44-53. doi: 10.11975/j.issn.1002-6819.202305243 WANG Kai, LIU Hanyu, WANG Like, et al. Effects of particle volume concentration on the leakage vortex and erosion characteristics of semi-open centrifugal pumps[J]. Transactions of the Chinese Society of Agricultural Engineering (Transactions of the CSAE), 2023, 39(16): 44-53. (in Chinese with English abstract) doi: 10.11975/j.issn.1002-6819.202305243
[3] LI G Y, DING X H, WU Y B, et al. Liquid-vapor two-phase flow in centrifugal pump: Cavitation, mass transfer, and impeller structure optimization[J]. Vacuum, 2022, 201: 111102. doi: 10.1016/j.vacuum.2022.111102
[4] 赵伟国,张栩,杨良,等. 凹槽结构对离心泵空化特性的影响[J]. 华中科技大学学报(自然科学版),2024,52(7):139-144. ZHAO Weiguo, ZHANG Xu, YANG Liang, et al. Influence of cavitation characteristics of groove structure for centrifugal pump[J]. Journal of Huazhong University of Science and Technology (Natural Science Edition), 2024, 52(7): 139-144. (in Chinese with English abstract)
[5] 阮辉,廖伟丽,罗兴锜,等. 叶片低压边的轴面位置对高水头水泵水轮机空化性能的影响[J]. 农业工程学报,2016,32(16):73-81. doi: 10.11975/j.issn.1002-6819.2016.16.011 RUAN Hui, LIAO Weili, LUO Xingqi, et al. Effects of low pressure meridional position on cavitation performance for high-head pump-turbine[J]. Transactions of the Chinese Society of Agricultural Engineering (Transactions of the CSAE), 2016, 32(16): 73-81. (in Chinese with English abstract) doi: 10.11975/j.issn.1002-6819.2016.16.011
[6] YUAN Z Y, ZHANG Y X, ZHANG J Y, et al. Experimental studies of unsteady cavitation at the tongue of a pump-turbine in pump mode[J]. Renewable Energy, 2021, 177: 1265-1281. doi: 10.1016/j.renene.2021.06.055
[7] LI D Y, ZHU Y T, LIN S, et al. Cavitation effects on pressure fluctuation in pump-turbine hump region[J]. Journal of Energy Storage, 2022, 47: 103936. doi: 10.1016/j.est.2021.103936
[8] MOUSMOULIS G, KASSANOS I, AGGIDIS G, et al. Numerical simulation of the performance of a centrifugal pump with a semi-open impeller under normal and cavitating conditions[J]. Applied Mathematical Modelling, 2021, 89: 1814-1834. doi: 10.1016/j.apm.2020.08.074
[9] 陆国成,左志钢,刘树红,等. 压水室形状对某离心泵径向力影响的数值模拟[J]. 工程热物理学报,2015,36(4):770-774. LU Guocheng, ZUO Zhigang, LIU Shuhong, et al. Numerical analysis of the influence of casing's shape on radial force of a centrifugal pump[J]. Journal of Engineering Thermophysics, 2015, 36(4): 770-774. (in Chinese with English abstract)
[10] 王悦荟. 核主泵入流畸变特性及其控制方法研究[D]. 杭州:浙江大学,2021. WANG Yuehui. Research on the Characteristics of Distortion Inflow and its Control Method on Reactor Coolant Pump with Steam Generator[D]. Hangzhou: Zhejiang University, 2021. (in Chinese with English abstract)
[11] ADAMKOWSKI A, HENKE A, LEWANDOWSKI M. Resonance of torsional vibrations of centrifugal pump shafts due to cavitation erosion of pump impellers[J]. Engineering Failure Analysis, 2016, 70: 56-72. doi: 10.1016/j.engfailanal.2016.07.011
[12] ZHAO G S, LIANG N, LI Q Q, et al. Effect mechanisms of leading-edge tubercle on blade cavitation control in a waterjet pump[J]. Ocean Engineering, 2023, 290: 116240. doi: 10.1016/j.oceaneng.2023.116240
[13] 孙龙刚,郭鹏程,郑小波,等. 混流式水轮机叶道空化涡诱发高振幅压力脉动特性[J]. 农业工程学报,2021,37(21):62-70. doi: 10.11975/j.issn.1002-6819.2021.21.008 SUN Longgang, GUO Pengcheng, ZHENG Xiaobo, et al. Characteristics of high-amplitude pressure fluctuation induced by inter-blade cavitation vortex in Francis turbine[J]. Transactions of the Chinese Society of Agricultural Engineering (Transactions of the CSAE), 2021, 37(21): 62-70. (in Chinese with English abstract) doi: 10.11975/j.issn.1002-6819.2021.21.008
[14] 朱国俊,李康,冯建军,等. 空化对轴流式水轮机尾水管压力脉动和转轮振动的影响[J]. 农业工程学报,2021,37(11):40-49. doi: 10.11975/j.issn.1002-6819.2021.11.005 ZHU Guojun, LI Kang, FENG Jianjun, et al. Effects of cavitation on pressure fluctuation of draft tube and runner vibration in a kaplan turbine[J]. Transactions of the Chinese Society of Agricultural Engineering (Transactions of the CSAE), 2021, 37(11): 40-49. (in Chinese with English abstract) doi: 10.11975/j.issn.1002-6819.2021.11.005
[15] LV Y, LI S, WANG Z, et al. Study on pump mode cavitation characteristic of variable speed pump turbine[J]. Physics of Fluids, 2023, 35(6): 065111. doi: 10.1063/5.0154131
[16] LIU Y, GONG J, AN K, et al. Cavitation characteristics and hydrodynamic radial forces of a reversible pump–turbine at pump mode[J]. Journal of Energy Engineering, 2020, 146(6): 04020066. doi: 10.1061/(ASCE)EY.1943-7897.0000713
[17] SUN W H, TAN L. Cavitation-vortex-pressure fluctuation interaction in a centrifugal pump using bubble rotation modified cavitation model under partial load[J]. Journal of Fluids Engineering, 2020, 142(5): 051206. doi: 10.1115/1.4045615
[18] 吴登昊,吴振兴,周佩剑,等. 低比转速离心泵叶轮瞬态空化特性分析[J]. 水力发电学报,2018,37(3):96-105. doi: 10.11660/slfdxb.20180311 WU Denghao, WU. Zhenxing, ZHOU Peijian, et al. Transient characteristics of cavitation in low specific speed centrifugal pumps[J]. Journal of Hydroelectric Engineering, 2018, 37(3): 96-105. (in Chinese with English abstract) doi: 10.11660/slfdxb.20180311
[19] ZHU D, XIAO R F, LIU W C. Influence of leading-edge cavitation on impeller blade axial force in the pump mode of reversible pump-turbine[J]. Renewable Energy, 2021, 163: 939-949. doi: 10.1016/j.renene.2020.09.002
[20] 李琪飞,张正杰,李仁年,等. 水泵水轮机泵工况空化特性与转轮受力分析[J]. 农业机械学报,2018,49(1):137-142. doi: 10.6041/j.issn.1000-1298.2018.01.017 LI Qifei, ZHANG Zhengjie, LI Rennian, et al. Analysis of cavitation performance and force on runner of pump-turbine in pump mode[J]. Transactions of the Chinese Society for Agricultural Machinery, 2018, 49(1): 137-142. (in Chinese with English abstract) doi: 10.6041/j.issn.1000-1298.2018.01.017
[21] HAO Y, TAN L. Symmetrical and unsymmetrical tip clearances on cavitation performance and radial force of a mixed flow pump as turbine at pump mode[J]. Renewable Energy, 2018, 127: 368-376. doi: 10.1016/j.renene.2018.04.072
[22] GONG B, ZHANG Z C, FENG C, et al. Experimental investigation of characteristics of tip leakage vortex cavitation-induced vibration of a pump[J]. Annals of Nuclear Energy, 2023, 192: 109935. doi: 10.1016/j.anucene.2023.109935
[23] 高波,杨敏官,李忠,等. 空化流动诱导离心泵低频振动的实验研究[J]. 工程热物理学报,2012,33(6):965-968. GAO Bo, YANG Minguan, LI Zhong, et al. Experimental study on cavitation induced low frequency vibration in a centrifugal pump[J]. Journal of Engineering Thermophysics. 2012, 33(6): 965-968. (in Chinese with English abstract)
[24] 叶阳辉,朱相源,孙光普,等. 离心泵内空泡演化与其对振动的影响[J]. 农业机械学报,2017,48(6):88-93,137. YE Yanghui, ZHU Xiangyuan, SUN Guangpu, et al. Evolution of cavitation bubbles and its influence on vibration in centrifugal pump[J]. Transactions of the Chinese Society for Agricultural Machinery, 2017, 48(6): 88-93,137. (in Chinese with English abstract)
[25] JIA X Q, YANG X Q, ZHU Z C. Study on pressure pulsations and transient fluid forces in centrifugal pump under different degrees of cavitation[J]. Proceedings of the Institution of Mechanical Engineers, Part E: Journal of Process Mechanical Engineering, 2024, 238(6): 2834-2844.
[26] CAO W D, JIA Z X, ZHAO Z J, et al. Validation and simulation of cavitation flow in a centrifugal pump by filter-based turbulence model[J]. Engineering Applications of Computational Fluid Mechanics, 2022, 16(1): 1724-1738. doi: 10.1080/19942060.2022.2111363
[27] SURYAWIJAYA P, KOSYNA G. Schaufeldruckmessungen im rotierenden System an einer Radialpumpe bei Gas/Flüssigkeitsgemischförderung[J]. Chemie Ingenieur Technik, 2000, 72(11): 1366-1371. doi: 10.1002/1522-2640(200011)72:11<1366::AID-CITE1366>3.0.CO;2-E
[28] FROBENIUS M, SCHILLING R, FRIEDRICHS J, et al. Numerical and experimental investigations of the cavitating flow in a centrifugal pump impeller[C]. Proceedings of the ASME 2002 Joint U. S. -European Fluids Engineering Division Conference, Quebe, Canada, 2002, 36150: 361-368.
[29] LU J X, LIU J H, QIAN L Y, et al. Investigation of pressure pulsation induced by quasi-steady cavitation in a centrifugal pump[J]. Physics of Fluids, 2023, 35(2): 025119. doi: 10.1063/5.0135095
[30] SCHNERR G H, SAUER J. Physical and numerical modeling of unsteady cavitation dynamics[C]. Fourth international conference on multiphase flow, New Orleans, USA, 2001, 1: 1-12.
下载:
计量
- 文章访问数: 119
- HTML全文浏览量: 12
- PDF下载量: 34
